ENG - Theory - The Atmosphere

The Sky and the Balloon

International Standard Atmosphere

To know how much a balloon can climb first we need to understand where it is climbing: The atmosphere.

There are many points of view to describe the Atmosphere, its layers, etc... So let's use a simple model known as the ISA model.

ISA = International Standard Atmosphere.

The ISA model is the one used in aeronautics and I think it will give us a simple image of the way to follow the Rockoon during the ascent beyond 100 km of altitude.

According to the ISA model the layers are:

The Troposphere

The Stratosphere

The Mesosphere

The Thermosphere

The Exosphere

Consecutively separated by the Tropopause, the Stratopause, the Mesopause and the Thermopause.

The Troposphere is approximately 10 to 20 km in height depending on latitude and other factors and where the temperature can drop to -56.5 ° C or lower.

The Stratosphere continues, reaching up to 50 km where curiously the temperature rises again uniformly until reaching about -3ºC.

Subsequently the Mesosphere, where the temperature descends again to -80ºC.

The thermosphere where temperatures can fluctuate between -80 ° C to 1500 ° C if solar activity is important. In the Thermosphere there is an imaginary line known as Kármán line, 100 km high and this is the goal in this adventure.

This line is considered the border between atmosphere and the outer space by the International Aeronautical Federation. And for the organizers of the N-prize is the minimum altitude that has to be reach by the femto-satellite.

At the topwe find the Exosphere that is already stable because it is practically empty. For us this layer goes out of rank of our interests.

The Trosposphere is the zone with the highest traffic density of all. It is also where practically all the humidity of the atmosphere is found, that implies that it is where practically all the atmospheric phenomenas develop.

It is highly recommended to avoid the Troposphere, used only for transit purposes.

Troposphere versus Stratosphere. source: theozonehole.com

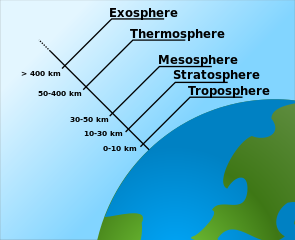
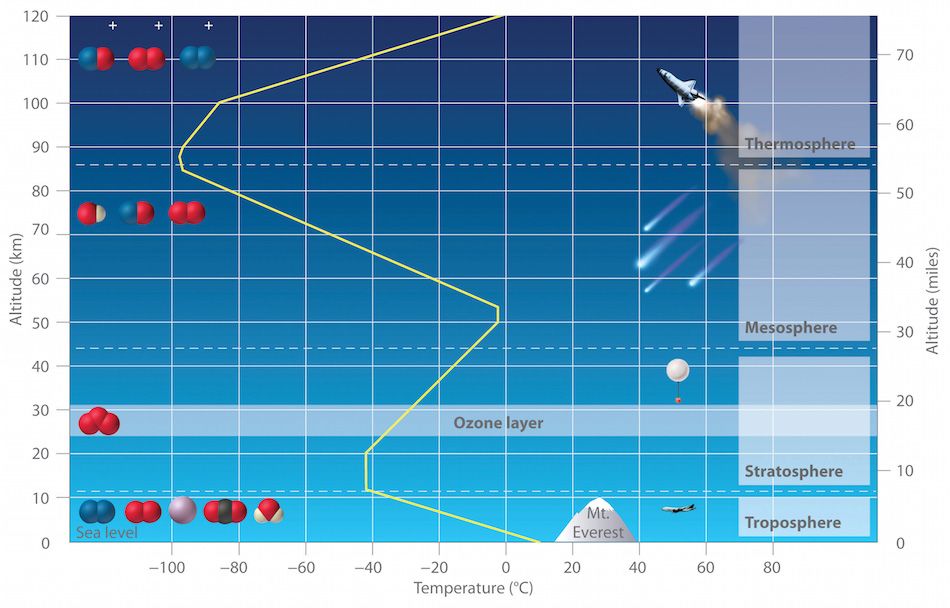
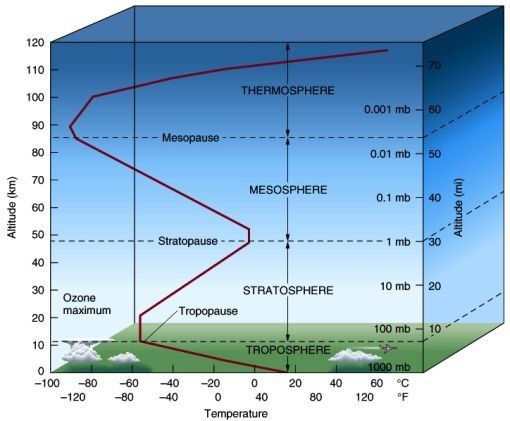
Now the question is, how can I avoid the Troposphere? Flying over it of course! and how can I do it? Lets see:

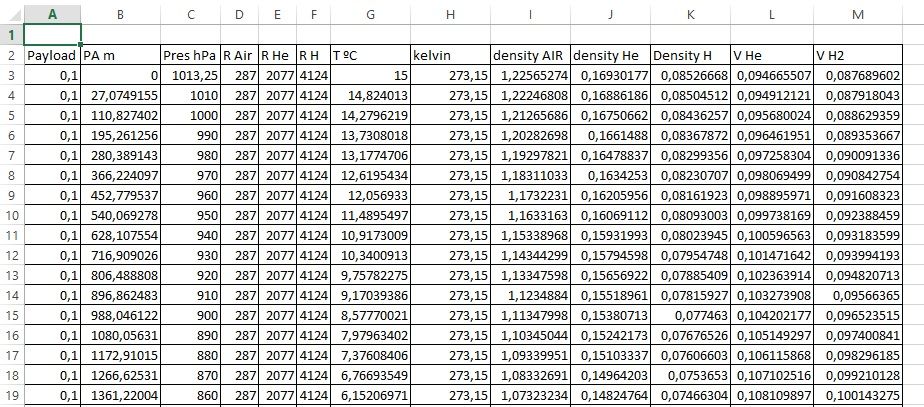
In case of a balloon, knowing the maximum volume, the weight of a balloon and its payload we can find out how to reach the point is know as Apogee.

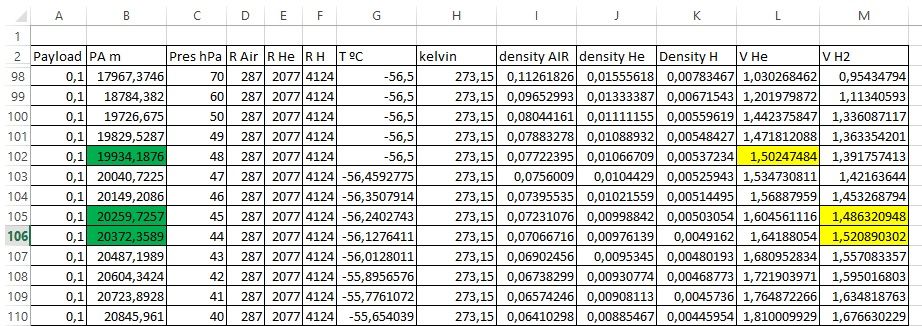
Now we are going to calculate the theoretical apogee ...remember I've said theoretical.

First we need to know the Temperature and Pressure, this two values are changing during the balloon climb.

The temperature in ISA atmosphere starts at Sea level at 15ºC and falls linearly until -56.46 ºC. We are able to know the decrease in temperature using the formula

Temp in celsius = 15 - (altitude in meters *0.0065)

At 11km altitude the temp is - 56.46ºC and then it keeps constant until the 20km or 25km depending the sources, after that rises linearly again until the Stratopause.

An approximate formula ( I use 20 km that is why is 20000 in the formula)

Temp in celsius = -56.5 + ((altitude in meters - 20000) * 0.001)

The temperature stops increasing at Stratopause and falls again in mesosphere and increases again in thermosphere.

Pressure never rise again but decreases in an exponential formula.

There are many websites in internet where you can find the formula of Pressure but I prefer to find altitude knowing the pressure. This formula relates each altitude at a given pressure.

Altitude = 8430.153 * LogN (1013.25/pressure))/(1+(0.095*LogN(1013,25/pressure))

As I said, there are some other formulas depending of the source, if you prefer to use the NASA formulas you can find here.

NASA's Earth Atmosphere Model in Imperial units

NASA's Earth Atmosphere Model in Metrical units

Great! We know pressure and temperature at a specific altitude. Now we can link with the last post, Archimedes Density and Volume.

Density = Pressure in Pa / (Gas constant R * Kelvin temperature)

Volume in liters = payload kg / (Density of air - density of gas used)

At this point we can prepare a Table with the formulas, for example using Excel or Calc program. Decreasing pressure we can find the Pressure Altitude, with PA we can find Temperature and then Densities and Volume of each gas.

Now imagine you have a balloon and its maximum volume is 1.5 m3, it weights 80 grams and your payload is 20 grams, that means a total weight of 0.1 kg.

As you can see in the table, the minimum volume of gas at sea level would be at least:

Density of Air = 1013.25 *100 / ( 286.9 * (15+273.15)) = 1.22565274 kg/m3

Density of H2 = 1013.25 *100 / ( 4124 * (15+273.15)) = 0.08526668 kg/m3

Density of He = 1013.25 *100 / ( 2077 * (15+273.15)) = 0.094665507 kg/m3

Volume of H2= 0.1 / (1.22565274 - 0.08526668) = 0.087689602 m3

Volume of He= 0.1 / (1.22565274 - 0.16930177) = 0.094665507 m3

We need to fill 88 liters of H2 or 95 liters of He.

At the moment we know how much H2 or He we need.

And knowing the balloon capacity is 1.5m3 we can look for the altitude.

The altitude is around 20km in both cases. As you can see the "performances" of He or H2 are very similar.

BACK