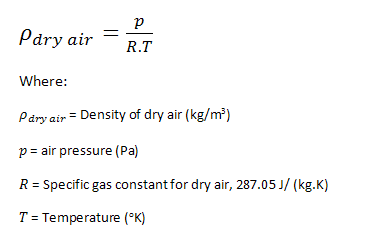There are basically two forces on the balloon.
One downward, the weight:
Weight = Mass * Gravity
The mass of the whole balloon, is the payload, the ropes, the wrapping of the balloon and so fort so on... ah! And the mass of the gas we are using, which even being lighter than air has also a mass.
For this example we assume that the balloon plus the load strings etc has a mass of 1 kg. The mass of the gas will depend on the volume so we will treat it separately.
At Gravity we will call it "g" and is a constant value of 9.8 m / s²
To the force Weight donwards we will call it "W".
And another up, the "push up" or ascensional force that we will call "P".
Archimedes' principle states that every body submerged in a fluid experiences a vertical and upward thrust equal to the weight of fluid dislodged. The "Push up" is therefore the weight of the dislodged fluid.
Push = Weight to be dislodged = density * Volume * Gravity
In order to rise a balloon, P must be greater than W:
P > W
Now some technical data (these data are extracted from Wikipedia, at the end will know how to calculate them)
Density of air 1.29 kg / m³
Density of Helium 0.1778 kg / m³
Density of Hydrogen 0.0838 kg / m³
(Densities measured at 1013.25 mb and 0°C)
As we have said we have a balloon of 1 kg of mass and we want to use Helium to make it rise. What volume of gas do we need?
We apply the principle of Archimedes.
"Da" will be the density of the air, "Dhe" the helium one.
Da = 1.29 kg / m³
Dhe = 0.18 kg / m³
Let's first calculate the Weight and then the Push
Here it is the unknown that we want to solve and we will call it V, this is the volume of gas we will need to achieve the "Push up", and then we will add a mass of 1 kg payload.
Weight = Mass * Gravity, and measured in Newtons
The gravity will be called "g" with a constant value of 9.8 m / s²
Therefore the weight without gas is:
W1 = 1 * 9.8
The principle of Archimedes states that
W = Dhe * g * V
The required Helium gas weight is:
W2 = 0.18 * 9.8 * V
Our balloon weight W is the W1 weight without gas + W2 the gas weight
W = (1 * 9,8) + (0,18 * 9,8 * V)
Now we have to know that the value of V, for that we use the same formula to know P
P = weight of the dislodged air (Archimedes principle) = Da * g * V
The dislodged gas is air therefore:
P = 1.29 * 9.8 * V
As it will have to be that P > W,
1.29 * 9.8 * V > (0.18 * 9.8 * V) + (1 * 9.8)
As you can see gravity does not affect the result and we can reduce the formula to:
1.29 * V > (0.18 * V) + 1
Or:
1.29 * V - 0.18 * V > 1
1.11 V > 1
That is the same
Thus
V > 1 / 1.11
V > 0.901 m³ ie 901 liters Helium
In short, as an special formula for our purpose we can use
V liters = payload kg / (Density of air - density of gas used)
Another example: if we use the Hydrogen and 1 kg of charge would look like this:
V = 1 / 1.29 - 0.084
V = 0.83 m³ ie 830 liters of H2
Although Helium duplicates the weight of Hydrogen, the difference in volume is only 8% in this example.
Another idea: can I use the same gas, inside and outside the balloon? (we're talking about air).
Yes, if we change the density of air inside the balloon.
The density data can be calculated using the formula of the ideal gas law, thans again to Wikipedia we know:
Density = Pressure in Pa / (Gas constant R * Kelvin temperature)
Thus, density varies with the pressure and with the temperature.
A solar balloon is the cheapest method to fly in a balloon, making the air inside the balloon less dense than outside one and using the Sun as a heater (but it works daylignt only).
According to Wikipedia the hot air at 50ºC has a density of 1.09 so to lift a kg we need:
V = 1 / 1.29 - 1.09
V = 1 / 0.2 = 5 m³ ie 5000 liters of Hot air at 50°C (assuming the outside air at 0°C).
As you can see, density is very important to calculate the weight of a gas.
and more, we have now a formula to calculate Density:
As I said, the density of a gas can be calculated using the ideal gas law, expressed as a function of temperature and pressure.
For example dry air: